Charge density study of tetrazole

L. Kucková, P. Herich, J. Kožíšek

Department of Physical Chemistry, Slovak University of Technology, 812 37 Bratislava, Slovakia

lenka.kuckova@stuba.sk

Introduction

Comparison of experimental and theoretical electronic structure is very important. In many cases the number of atoms of the studied system make difficulties. In this work tetrazole was carefully chosen as the smallest single molecule in the unit cell. This work deals with the study of experimental electronic structure of tetrazole.

Tetrazole and its derivatives have attracted considerable attention because of their characterful structure and their applications as anti-allergic, antihypertensive, antibiotic and anticonvulsant agents. Therefore it plays an important role in medicinal chemistry. Tetrazoles, as quite suitable ligands, can serve as replacement for carboxylic acid also in supramolecular chemistry. Moreover, tetrazoles are highly flexible ligands and can easily adapt to different binding modes [1-5].

Experimental

Tetrazole was purchased from Sigma Aldrich as a solution for reaction. After the solvent was vaporized, the crystals were prepared by slow crystallization from the mixture of ethanol – isobutanol (6:1).

A single crystal of tetrazole was selected and mounted in the cold nitrogen stream. The data were collected at 100.0 (1) K on an Oxford Diffraction Kappa geometry GEMINI R diffractometer equipped with Ruby CCD area detector using graphite monochromated MoKα radiation (λ=0.71073 Å) at 50 kV and 40 mA. Distance from crystal to detector was 53 mm. Details of the X-ray diffraction experiment conditions and the crystallographic data for tetrazole are given in Tab. 1. Crystal structure was solved and refined by using SHELXS-97 and SHELXL-97. The molecular structure of tetrazole and its perspective view are shown in Fig. 1.

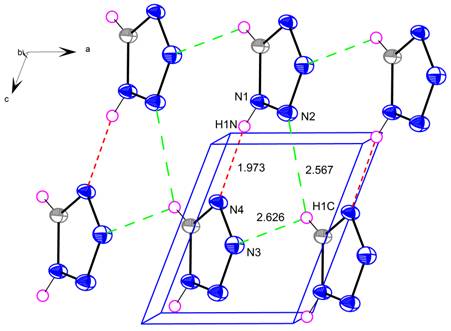
Figure 1. A perspective view of tetrazole (ellipsoids are drawn in 50 % probability factor). Contact distances and hydrogen bonds are shown by dotted lines.

Starting parameters for multiple refinement were taken from a routine SHELXL refinement and all other refinements were carried out on F using the XD suite of programs [6]. A complete atom-centered multipole refinement was carried out with the nonspherical atomic electron density given by the equation (1) [7].

r_at(r) = P_c r_core(r) + P_v k³ r_valence(kr) + k’³ R_l(k’r) P_lm±d_lm±(q,j) (1)

The H atoms were treated with one bond-directed dipole (l = 1), other atoms were refined up to octapoles. The local coordinate systems to define multipoles were used as follows. For non-hydrogen atoms: x-axis - direction to the closest atom, y-axis - perpendicular to the x-axis and oriented towards the second closest atom; for hydrogen atoms: z-axis - direction to the bonding carbon or nitrogen atom and x-axis - perpendicular to the z-axis. The strategy for refinement was as described previously [6]. The results of refinement are summarized in Tab. 2.

Table 1. Crystal data and experimental details for tetrazole.

Empirical formula	C H2 N4	Crystal size	0.264 x 0.162 x 0.058 mm
Formula weight	70.07	θ range for data collection	4.68° to 45.59°
Temperature, wavelenght	293 (2) K, 0.71073 Å	Index ranges	-7<=h<=6
Crystal system, space group	triclinic, P 1		-9<=k<=9
Unit cell dimensions	a = 3.6064 (4) Å		-9<=l<=9
	b = 4.7373 (6) Å	Max. and min. transmission	0.994 and 0.977
	c = 4.9287 (9) Å	Reflections collected	5398
	α = 107.1 (1)°	Independent reflections	1961 (R(int) = 0.0333)
	β = 107.8 (2)°	Completeness to 2θ = 25.00	100%
	γ = 100.1 (1)°	Data / restraints / parameters	1961 / 3 / 46
Formula units per unit cell	1	Goodness-of-fit on F^2 1.041	1.041
Calculated density	1.589 mg m^-3	Final R indices [I>σ(I)] R1 = 0.0388, wR2 = 0.0984	R1 = 0.0388, wR2 = 0.0984
Absorption coefficient	0.124 mm^-1	R indices (all data)	R1 = 0.0434, wR2 = 0.1034
F (000)	36	Largest diff. peak and hole 0.349 and -0.404 (eÅ^-3)	0.349 and -0.404 (eÅ^-3)

As can be seen in Table 2, the multipole refinement resulted in a significant improvement of the agreement between the experimental and calculated structure factors. Residual density maps were calculated by a Fourier synthesis where the coefficients are differences between the observed and calculated structure factors corresponding to the converged multipole model.

Table 2. Summary of the SHELXLand multipole refinement of tetrazole.

	SHELXL refinement	Multipole refinement
R(F)	-	0.0188
R(F)^†	-	0.0191
wR(F)^†	-	0.0148
R(F^2)	0.0388	0.0241
R(F^2)^†	0.0434	0.0242
wR(F^2)^†	0.1034	0.0300
S	1.041	1.4455

The maximum and minimum of the residual density are +0.108 e/ų and −0.074 e/ų, respectively; the root-mean-square residual density is 0.034 e/ų. Atoms in ring are bonded by covalent bonds. Inspection of the maximum charge concentrations in the bonding and nonbonding regions in the valence shell, the so-called valence shell charge concentrations (VSCCs) shows that there are three charge concentrations (Fig. 2), which correspond to lone electron pairs on nitrogen atoms. On the other hand, the depletion of the charge in the regions where the covalent bonds are formed by interaction with the lone pair on carbon and nitrogen donor atoms (C(1), N(1), N(2), N(3) and N(4)) is clearly seen (Fig. 2).

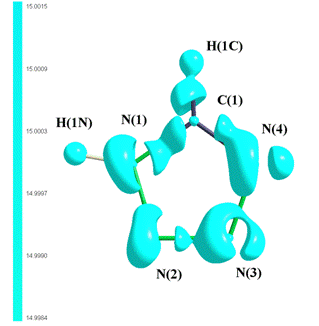
Figure 2. 3D plots of the Laplacian of the electron density in tetrazole.

References

1. R. N. Butter, in Comprehensive Heterocyclic Chemistry, A. R. Katritzky, C. W. Rees, Eds., Vol. 5, Part 4A, Pergamon Press, New York, 1984, p. 791

2. S. Bergmans, J. Hunt, A. Roach, P. Goldsmith, Epilepsy Res. 75 (2007) 18

3. J. H. Toney, P. M. D. Fitzgerald, N. Grover Sharma, S. H. Olson, W. J. May, J. G. Sundelof, D. E. Venderwall, K. A. Cleary, S. K. Grant, J. K. Wu, J. W. Kozarich, D. L. Pompliano, G. G. Hammond, Chem. Biol. 5 (1998) 185

4. L. V. Myznikov, A. Hrabalek, G. I. Koldobskii, Chem Heterocycl. Compd. 43 (2007) 1

5. H. D. Klaubert, J. H. Sellstedt, C. J. Guinosso, S. C. Bell, R. J. Capetola, J. Med. Chem. 24 (1981) 748

6. Koritsanszky, T.; Howard, S.T.; Su, Z.; Mallinson, P.R.; Richter, T.; Hansen, N.K. (1997), XD, Computer Program Package for Multipole Refinement and Analysis of Electron Densities from Diffraction Data. Free University of Berlin, Germany.

7. Coppens, P. X-ray Charge Densities and Chemical Bonding, Oxford University Press, 1997.

Acknowledgements.

This work has been supported by Slovak Grant Agency APVV (APVV-0202-10) and VEGA (1/0679/11).