Squarefree numbers and their diffraction
Michael Baake, Christian Huck and Tobias Jakobi
Department of Mathematics, University of Bielefeld (Germany)
{mbaake,huck,tjakobi}@math.uni-bielefeld.de
An integer is called squarefree if it is not divisible by a nontrivial square. The set of squarefree integers is a discrete subset of the line with gaps of arbitrary size. Nevertheless, it has positive density and a pure point diffraction spectrum [1, 2], as well as other interesting properties as a dynamical system [3, 4].
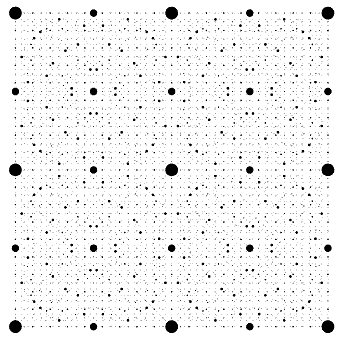
Figure 1. Diffraction of
the squarefree Gaussian numbers (non-linear scaling of intensities).
Recently, the setting was generalized [5] to squarefree numbers in algebraic number fields, where many properties prevail. In this contribution, which complements the tutorial talk [6], some explicit examples are shown in detail. Our emphasis is on the connection with the underlying Minkowski embedding [5].
In particular, we present the diffraction for the squarefree numbers in various rings of integers of quadratic number fields, including the Gaussian integers Z[i] as well as Z[Ö2] and Z[t], where t is the golden ratio.
1. M. Baake, R. V. Moody, P. A. B. Pleasants, Diffraction from visible lattice points and k-th power free integers, Discr. Math., 221 (2000), pp. 3-42.
2. M. Baake, U. Grimm, Aperiodic Order. Vol. I: A Mathematical Invitation. Cambridge University Press, Cambridge. 2013.
3. F. Cellarosi, Y. G. Sinai, Ergodic properties of
square-free numbers, J. Eur. Math. Soc., 15 (2013),
pp. 1343-1374.
4. C. Huck, M. Baake, Dynamical properties of k-free lattice points, Acta Phys. Pol., A126 (2014), pp. 482-485.
5. F. Cellarosi, I. Vinogradov, Ergodic properties of k-
free integers in number fields. J. Mod. Dynam., 3 (2013),
pp. 461-488.
6. C. Huck, Visible lattice points and weak model sets, Aperiodic 2015 tutorial talk.
This work was supported by the German Research Foundation (DFG) within the CRC 701.